

| How To Avoid Nearsightedness
|
It seems that the human mind has first to construct forms independently before we can find them in things. Kepler's marvelous achievement is a particularly fine example of the truth that knowledge cannot spring from experience alone, but only from the comparison of the inventions of the mind with observed fact. - Albert Einstein
Critical experiments are those experiments that allow us to choose between two major versions of factual truth. Without this check of physical reality, we can never determine the behavioral characteristic of the normal eye. The concept that the normal eye is a rigid system is potentially a valid concept -- until we actually make measurements of the impact that a confined environment has focal state of the normal eye. When we make the measurements, we find that the Helmholtz-passive theory is not accurate in accounting for the experimental data.
The normal human and primate eye maintain a high degree of focal accuracy while major optical components change in an unpredictable manner. (1) The equation, developed from a dynamic model that is capable of accounting for this degree of accuracy, also predicts that the eye's focal status will display a time-constant effect to a step change in its visual environment. (2) (3) The predictions of this theory are compared on a qualitative and semi-quantitative basis with a Helmholtz-passive theory of the normal eye's behavior.
While such a test cannot be carried out on humans, monkeys can be subjected to a step-change in their visual environment. (4) The following equation predicts the eye's focal status as a function of time:
Focus = Offset + Accommodation + Delta * [1 - EXP( -t/TAU ) ]
The required values are the average value of accommodation before and after the start of the test.
The time-constant, TAU, has an approximate value of 100 days for pigtail macaque monkeys. The physiological offset is a measurable characteristic of the human and primate eye. It has an approximate value of +1.5 diopters. Further experiment and measurement will be required to establish greater accuracy for these fundamental constants of the normal eye's behavior.
The average value of accommodation is determined by the visual environment of the eye. For instance, if a monkey is kept in a hooded visual environment of 20 inches, his environment will have a minimum value of -2 diopters. If he spends 50 percent of his time looking at 20 inches (-2 diopters), and the other 50 percent of his time looking at 12 inches (-3.2 diopters), his average value of accommodation will be -2.6 diopters. Alternatively, if he spends 100 percent of his time looking at 15 inches (-2.6 Diopters), his visual environment will be -2.6 Diopters.
By this technique of quantitative estimation, and by actual observational measurements, we can establish the average value of accommodation for monkeys kept in various visual environments. The following values are preliminary estimates:
| WILD | CAGED | HOODED |
| - 0.8 Diopters | -1.8 Diopters | - 2.6 Diopters |
If monkeys in a caged visual environment are placed in a hooded environment, their eyes will experience a step-change of:
1.8 - 2.6 = - 0.8 Diopters
Before the start of the test the focal status is: (At t = 0 )
Focus = 1.5 + (-1.8) + (0) * [ 1 - EXP ( - 0 / 100 ) ]
Focus = -0.3 Diopters
After 294 days their focal status will be:
Focus = 1.5 + (-1.8) + (-0.8) * [1 - EXP ( - 294 / 100 ) ]
Focus = - 1.1 Diopters
Dr. Young used Macaca Nemestrina (Pigtail) monkeys in his test. The monkeys were placed in a chair with their heads situated so their maximum visual distance was limited. The hoods were not more than 20 inches from the eyes of the monkeys at the furthest point, and averaged around 14 inches.
Nine adolescent animals were selected and a control group was maintained. Their refractive status was measured at two week intervals. The experiment was continued for eleven months. The measured mean focal status for these monkeys is shown on the FORTRAN generated graph. Three monkeys were removed from the test after four months due to pregnancy and sickness.
The refractive characteristics of the control group did not exhibit the time-constant effect demonstrated by the monkeys subjected to a step change in their visual environment. (Figure 1)
(FIGURE 1) THIS GRAPH SHOWS THE PREDICTIONS OF TWO THEORIES
DAYS FEED- MEAS- DIOPTERS NEARSIGHTED
INTO BACK URED -1.1 -1.0 -.9 -.8 -.7 -.6 -.5 -.4 -.3 -.2 -.1
TEST ...................................................
0 -.33 -.33 <-----------------------------------<< M
7 -.38 Accommodation Delta F H
14 -.43 -.40 - 0.8 Diopters F M H
21 -.48 F H
28 -.53 -.48 F M H
35 -.57 F H
42 -.60 -.60 M H
49 -.64 F H
56 -.67 -.71 MF H
63 -.70 Measured (M) F H
70 -.73 -.78 Status >>---> M F H
77 -.76 F H
84 -.78 -.83 M F H
91 -.81 F H
98 -.83 -.87 M F H
105 -.85 F H
112 -.87 -.90 M F H
119 -.89 F H
126 -.90 -.95 M F H
133 -.92 F H
140 -.93 -1.00 M F H
147 -.95 F Test Group (F) H
154 -.96 -1.05 M F <-----<< Prediction H
161 -.97 F H
168 -.98 -1.10 M F H
175 -.99 F H
182 -1.00 -1.08 M F H
189 -1.01 F H
196 -1.02 -1.06 M F Control Group (H) H
203 -1.02 F Prediction >>--------> H
210 -1.03 -1.07 M F H
217 -1.04 F H
224 -1.04 -1.08 M F H
231 -1.05 F H
238 -1.06 -1.09 M F H
245 -1.06 F H
252 -1.07 -1.10 M F H
259 -1.07 F H
266 -1.07 -1.10 MF H
273 -1.08 F H
280 -1.08 -1.10 MF H
287 -1.08 F H
294 -1.09 -1.10 MF H
...................................................
-1.1 -1.0 -.9 -.8 -.7 -.6 -.5 -.4 -.3 -.2 -.1
There are two fundamental theories of how the normal eye sets its focus while growing. One theory can be described as a Helmholtz-heredity theory of the eye's focal growth. This theory states that the cause of nearsightedness is purely genetic in origin, and asserts that the visual environment has no effect on the focal state of the normal eye. This is a passive theory of the normal eye's behavior.
For this experiment the prediction of this theory is that there should be no change in the focal status of monkeys who experience a delta in their visual environment, since their genetic characteristic is not altered by the experimental situation. Alternatively, the prediction of this theory is that no difference in focal status should develop between the normal eyes of the test group relative to the control group.
A dynamic (feedback control) theory states that the eye continuously servos, or sets its focus based on the eye's average value of accommodation. This theory predicts that there will be a time-constant response to a delta in the eye's value of accommodation.
The best way to choose between these two competing theories is to compare their predictions on a qualitative and semi-quantitative basis. On a qualitative basis the dynamic theory predicts a net change in focal state of the test group relative to the control group. The passive theory predicts no change. The passive theory can not be put in a form which will yield quantitative predictions for the eye's focal status as a function of time, and for that reason cannot be compared on a quantitative basis.
The Laplace transfer function for the long-term focal control behavior of the normal eye is: (2)
The impulse (perturbation) time response of this function is: (5)
This time-domain equation represents the basic underlying dynamic behavior characteristic of the normal eye when it is subjected to a sudden change in its accommodation status.
If the estimated value of accommodation ( - .8 Diopters) for a population of wild monkeys is used in the impulse equation, the result is:
Focus = 1.5 + ( -.8 ) - ( 0 ) * EXP ( - t / TAU )
Focus = + 0.7 Diopters
The plus indicates that the normal eyes of these monkeys have a normal (positive) focal state. The focal status of the normal eye (hyperopia) is measured with a plus lens. The measurement for the normal eye's focal status is made with the individual reading the eye chart at 20/20. Increasingly stronger positive lenses are placed in front of the eye until a lens strong enough to blur the 20/20 line is obtained. This lens strength is the specific value for the focal state of the normal eye.
A positive focal state (sometimes called hyperopia) is the condition of the normal eye. If the eye is placed in a confined visual environment, the eye will gradually change its focal status in a negative direction. When the normal eye changes its focal state to a minus value the eye is said to be nearsighted. This result is observed in populations of Naval students. (6)
Initially, the monkeys in this experiment were, on the average, slightly nearsighted. Their eyes were 20/25 at the start of the test and became more myopic (20/80) at the end of the test. Wild monkeys have 20/20 vision with an average focal state of +0.7 diopters.
| Figure 2 The Correlation of the Eye's Focal Status to the Snellen Eye Chart (Approximate) |
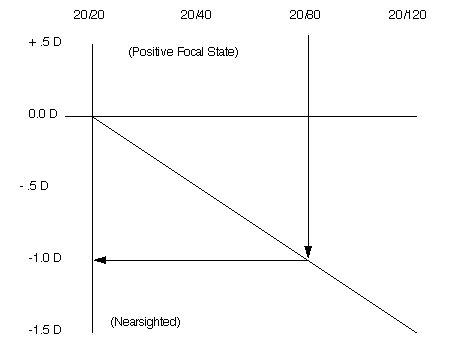 |
| If you read the chart at 20/80, you will normally receive a -1.0 diopter lens to match the problem. |
(See Fortran graph for data.)
| Unexplained Variation | = 0.07227 |
| Explained Variation | = 1.23809 |
| Total Variation | = 1.31037 |
| Correlation Coefficient | = 0.97203 |
This data, which represents the fundamental behavior characteristic of the normal eye, correlates with the equation:
Focus = Offset + Accommodation + Delta * [1 - EXP(-t/TAU ) ]
Was the correlation coefficient from this experiment accidental? Did Dr. Young randomly obtain 0.972 for the monkeys in the test when the actual population correlation coefficient was zero? This assertion can be checked by use of the students "t" distribution:
r
t = ------------------------
___________________
/
/ 1 - r2
\ / ---------
\/ n - 2
Where:
n = 23 (Number of measurements made)
r = 0.97 (Correlation coefficient from the experiment)
for v = 21 (Degrees of freedom = 23 - 2)
t.001 = 3.819 (Value for 99.9 percent confidence limit)
t = 0.97 / SQRT [ ( 1 - 0.972 ) / ( 23 - 2 ) ]
t = 18.28
Since 18.28 exceeds 3.819 (the 99.9 percent confidence limit) we can reject the idea that the Helmholtz-passive concept is correct. There is a very high correlation between the average value of accommodation and the focal state of the normal eye.
If the experiment is repeated 100 times, will we get the same correlation coefficient? What is the range of correlation coefficients that we can expect from the large population of normal eyed individuals?
If from a bivariate population with a correlation coefficient, RHO, all samples of size n are taken, then:
Z - m
(r) (RHO)
z = -----------------
Sigma
Where:
Z(r) = 0.5 * ln [ (1 + r) / (1 - r) ]
m(RHO) = 0.5 * ln [ (1 + RHO) / (1 - RHO) ]
Sigma = 1 / Square Root (n - 3)
z = Abscissa for area under probability curve
Values:
r = 0.97
Z(r) = 2.092
n = 23
Sigma = 0.2236
Z = +/- 2.58 for 99 percent confidence
Area = 1.0 - 2 * ( 0.495 ) = .01
By rearranging the equation:
m(RHO) = Z(r) +/- (Sigma * z)
using values: r = 0.97, n = 23, z = +/- 2.58
m(RHO) = 2.092 +/- 0.57688
Using look-up tables:
m(RHO) = 2.6688 and therefore the upper limit for RHO is 0.99
m(RHO) = 1.5151 and therefore the lower limit for RHO is 0.90
In other words, given the results of this experiment, we can conclude that it is virtually certain that the large-scale population coefficient will lie between 0.90 and 0.99 for all primate eyes.
There is a very high correlation between the normal eye's accommodation system and the focal state of the normal eye. The concept that the normal eye behaves as a (dynamic) neurological control system is strongly supported by direct factual data. The concept that the normal eye is passive in its behavioral characteristic is rejected by direct factual data.
These statistical tests are standard and conclusively demonstrate the truth that the normal eye DOES NOT obey the Helmholtz-passive model for the normal eye's behavior. It is very unlikely that future experiments will support the Helmholtz-passive model of the normal eye's behavior.
There are two powerful conceptual tools available for dealing with difficult servo problems -- analysis and synthesis. Since it is almost impossible to gain access to the accommodation system (that controls the eye's long-term focus), an indirect approach is required to establish the fundamental behavior characteristic of the normal human eye.
An indirect approach results in the development of mathematical models. By constructing two reasonable physiological models for the normal eye's behavior, we can develop two sets of theoretical predictions. We can then decide, on the basis of direct experimentation, which model is more fully confirmed by the available experimental evidence.
An analysis of the focal design requirements of the normal eye demonstrates that each eye must maintain a dynamic accuracy of better than 1.5 percent while growing to maintain normal vision. (5)
In synthesis, we develop a dynamic design which will account for the maximum number of facts known about the normal eye's focal setting action. Since the human body relies on feedback control principles in its design -- accommodation, temperature, and pH levels -- we find it appropriate to apply this concept to the eye's focal behavior. The opposite suggestion, that the normal eye ignores the accommodation signal while growing, leads to a theory that is incapable of accurate quantitative predictions.
This analysis/synthesis approach points to an equation that accurately predicts the dynamic behavior of the human and primate eye. The equation can support a procedure that will be effective in preventing nearsightedness, if the eye's dynamic behavior is understood, and the preventative procedure is assiduously carried out.
Accuracy and stability of the normal eye's behavior can be understood by modeling the eye as a servomechanism. An eye with this type of control system will exhibit a time-constant effect if subjected to a step-change in the eye's visual environment. In this experiment a "brute force" change was induced in the average visual environment. A time-constant response was measured in the eye's focal status. The theory which is compared to this concept is a Helmholtz-passive theory of the eye's focal behavior.
The dynamic analysis leads to a general equation for the long-term behavior of the normal eye. On the basis of this experiment we suggest that the dynamic (cybernetic) model is strengthened.
As with most mathematical models, certain effects (e.g., noise and perturbations in the system) have not been included. These effects will be assessed and represented in later chapters. Our experience, however, indicates that this model is very accurate with respect to other dynamic tests that have established the normal eye's behavioral characteristic.
In the absence of any other experimentally confirmed equation we can tentatively conclude that this test confirms the accuracy of this equation -- within the limits imposed by the experimental data that is available to us.
1. Young, F., Leary, G. VISUAL CHARACTERISTICS OF APES AND PERSONS, (207-225) Progress in Ape Research (1977)
2. Brown, O., Berger, R. A NEARSIGHTEDNESS COMPUTER, (343-346), The 7th Annual New England Bioengineering Conference (1979)
3. Brown, O., Young, F. PHYSIOLOGICAL MODELING: THE LONG-TERM GROWTH OF THE EYE, (133-136) The 8th Annual New England Bioengineering Conference (1980)
4. Young, F. THE EFFECT OF RESTRICTED VISUAL SPACE ON THE PRIMATE EYE, American Journal of Ophthalmology, Vol. 52, No. 5, Part II, November 1961
5. Brown, O., Young, F. THE RESPONSE OF A SERVO CONTROLLED EYE TO FOCAL PERTURBATIONS, The 2nd Annual conference of the IEEE Engineering in Medicine and Biology Society (1980)
6. Hayden, R. DEVELOPMENT AND PREVENTION OF MYOPIA AT THE UNITED STATES NAVAL ACADEMY, Archives of Ophthalmology Volume 25, #4 (April 1941)